by Lori McPherson, Iwaki America Corporation
An underused control strategy that offers significant benefits is Time-Proportional Control (TPC). Unlike traditional proportional or even PID control that require a varying output to a modulating control device, time-proportional control can achieve a proportional control response to process variation using an on/off device by varying on and off times in a defined control period. The on/off device is generally a simpler, less expensive control device.
First, a quick review of the first two common methods. Proportional analog is the most common control signal. This signal is often sent to a modulating control valve (positioner), variable speed pump (centrifugal or diaphragm metering), or to a variety of mechanical control options such as speed of a belt drive, etc. The premise is straightforward – the signal value from 4-20 mA creates a direct, proportional output response (0-100%) of the control device.
In basic proportional only control, the signal value is calculated from a linear relationship based on the variance from set-point with a maximum output set at a fixed maximum variance. A common example is in pH control where the output to an acid metering pump will vary from 4-20 mA as the pH increases from 8-10 pH. At 8 pH or less, 4 mA is sent to achieve 0% output of the pump. As the pH increases to 10 pH, the output will increase linearly, until at 10 or greater pH, 20 mA will be sent to the pump to achieve 100% output.
In more sophisticated control scenarios, the algorithm to determine the output value may incorporate Integral and possibly Derivative calculations (PI, or PID Control) to enable a more predictive output based on the speed of the process response to the output. Even with PI or PID control, the actual output signal remains a 4-20mA resulting in a proportional 0-100% response of the control device.
Proportional Pulse control is used specifically for proportional control of solenoid driven metering pumps. A controller varies a pulse output based on deviation from setpoint, similar to Proportional analog control. Using our same acid feed control example as above, the controller will send 0 pulses per minute to the metering pump at pH 8 or less, increasing the pulse/minute output proportional until at pH 10 or greater, the maximum pulse output will be generated. The metering pump will stroke one time for each pulse it receives, enabling the increase in pH to cause an increase in the volume output of the metering pump.
Time-Proportional Control
Time-Proportional control is a less widely used method for achieving proportional control, and has the advantage that it uses a lower cost on/off control device such as a solenoid valve, or fixed output pump. By proportioning the on-time vs. off-time of the control device within a fixed time period (sample period), a proportional response is achieved. The off-time portions of control provide an additional benefit by enabling better mixing of the process to occur, or time for reactions to take place.
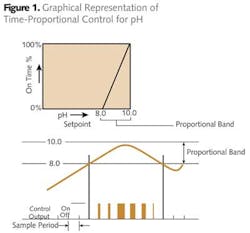
The parameters used to program a time-proportional output include the sample period, the set-point, the proportional band, and the control direction. The set-point is the desired pH of the system; while the control direction determines whether the output will increase above the set point (often called a High Set-Point) or below the set-point (Low Set-Point).
The sample period should be set to approximately 1½ times the amount of time that it takes for the system to react to the chemical addition. This can be determined by making a manual addition of chemical and timing how long it takes for the process to react. Setting the sample period too low will result in a second addition being made before the first is detected and will cause set point overshoot. Setting the sample period too high will delay the next addition and can prevent the set point being reached.
Finally, the Proportional Band is the deviation from set point that will result in a 100% on time of the output. Returning to our acid feed pump example for pH control, we could set a sample period of 10 minutes, a High Set-Point of 8.0, and a Proportional Band of 2 pH (from 8 to 10). At 8 pH or below, the output would be off 100% of the time. At pH 10.0 or above, the output would be on for 100% of the next 10 minute sample period. At 9 pH, the output would be on for 5 minutes, then off for 5 minutes. The percentage of on-time of the 10 minute sample period increases proportionally as the process moves away from the setpoint. The actual on-time can be calculated as follows:
The off-time will be the remainder of the Sample Period time.
In the Figure 1 example, the set point is 8.0 pH and the Proportional Band is 2.0 pH. Note that when the pH goes above the set point, the control relay is ON for a short period of time. As the pH increases, the control relay is ON for a longer period of time. When the addition starts to affect the process pH and the pH is reduced, the control relay is ON for a shorter period of time. When the pH drops below the set point of 8.0, the control relay is OFF all the time.
In addition to lower cost control devices, there can also be other process benefits. Some plants will use waste process streams as their neutralizing chemicals (i.e. waste acid baths as acid reagent, or waste cleaners (generally alkaline) as caustic reagents). Many of these streams are too dirty to be pumped using standard solenoid metering pumps. A solenoid or actuated ball or diaphragm valve on a gravity feed system can provide a lower maintenance option.
Time-proportional control should not be used in once through (on-the-fly) control processes, such as chemical injection into a pipe. These systems require feedback and prediction algorithms that are found in PID. Time-proportional control is well suited for recirculating processes, or those with a built in retention time (i.e. neutralization tank). A system with a retention time of 10 minutes or longer is suitable. To determine the retention time of a system, simply divide the tank volume by the flow through the tank, i.e. a 600 gallon tank with flow in/out at 60 gpm equals a 10 minute retention time.
Time-proportional control has been available for a long time, but is often overlooked by process control experts who tend to use more sophisticated PID algorithms, often with self-tuning or other enhancements, even when they're not necessary. While there is a place for these, the simpler time-proportional control algorithm should not be forgotten. It can provide simple, enhanced control using lower maintenance components to achieve improved control in many applications.
About the Author: Lori McPherson has been a Regional Sales Manager with Walchem for 14 years. She has a B.S. in Chemical Engineering from Purdue University, and a M.S. in Systems Engineering from Virginia Tech. Prior to Walchem she has held positions as a Process Engineer, Waste Treatment Engineer, and Analytical Product Manager specializing in Conductivity, pH and ORP Measurements. She has previously published papers focused on ORP measurement and control, and accurate conductivity measurements.